Reliable, low-cost, fully integrated hydration sensors for monitoring and diagnosis of inflammatory skin diseases in any environment

Device structure and operation

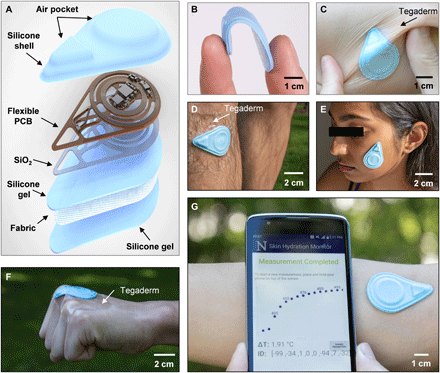
The SHS measures the thermal properties (conductivity k and diffusivity α) of the skin using the transient plane source (TPS) technique. TPS exploits joule heating via a resistive element placed on top of a sample of interest while simultaneously recording the transient temperature change (ΔT) of the heating element itself or of a separate temperature sensor (10). An exploded-view diagram of the SHS appears in Fig. 1A. The flexible printed circuit board (f-PCB) contains electronics for sensing and wireless communication. Open spaces/mechanical relief cuts in the polyimide (PI) layer of the f-PCB enhance the overall flexibility and limit lateral thermal transport through the PI, away from the sensing components. To ensure low thermal resistance to the skin, a thin bottom silicone gel adhesive (~120 μm thick) bonds by a covalent dehydration reaction between ─OH groups on the surface of the silicone to a thin layer of SiO₂ (~75 nm) sputter coated on the backside of the f-PCB. An ultrathin fabric of fiberglass (~30 μm) embedded in the silicone gel greatly enhances the mechanical robustness of the system. Repeated application and removal of the device are possible without irritation to the skin or damage to the device. Cleaning with alcohol wipes provides a convenient means to sterilize the device for use across different patients with minimal risk and restores the tackiness of the adhesive by eliminating particulates or other contaminants (sensors were tested for at least 130 times on average without damage). The shell-like top encapsulation layer creates small air gaps to thermally insulate the critical sensing components. This design also leads to low flexural rigidity and effective modulus, to facilitate application even on highly curved features of the body. Detailed fabrication procedures are in Materials and Methods, note S1, and fig. S1.

The resulting hydration sensors are flexible, thin (~2.1 mm), lightweight (~2.5 g), and have small lateral dimensions (~4.5 cm by 3.1 cm) (Fig. 1, B and C), enabling gentle yet intimate contact with the surface of the skin for application on nearly any region of the body, including challenging areas such as the shin, face, and even the knuckles (Fig. 1, D to F). The SHS wirelessly receives power from and communicates with any standard smartphone that has near-field communication (NFC) capabilities (Fig. 1G), thereby eliminating the need for a battery and allowing for a compact form factor. This collection of features suggests the potential for widespread use, not only in clinical and laboratory settings but also in the home or office. An easy-to-use, custom software application runs on the phone as an interface to allow patients to monitor their skin health and share data with their physician.

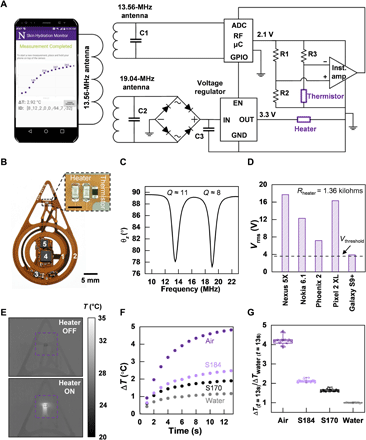
A detailed circuit diagram for the f-PCB electronics appears in Fig. 2A. Accurate measurements require relatively high levels of heating power (~10 to 60 mW) (7). The transmission coil of the smartphone serves as the primary 13.56-MHz (standard NFC frequency) antenna. The sensor design reported here involves separate power-harvesting coil antennas for the heating- and temperature-sensing circuits, in a concentric geometry (Fig. 2, A to C). The secondary antenna 1 (Ant. 1), tuned to 13.56 MHz, connects to the radio frequency (RF) microcontroller (μC) for powering the sensing circuit and for communicating data to the phone using NFC protocols. The secondary antenna 2 (Ant. 2), tuned to 19.04 MHz to prevent interference with Ant. 1, powers the heater (Fig. 2C). The quality factor (Q) of Ant. 1 is relatively high (~11) to enable good communication distance and coupling across phones with different primary antennae. The Q for Ant. 2 is relatively low (~8) to support adequate power harvesting despite the difference between its resonance frequency and that of the primary coil. Detailed antenna characteristics and computational results on the interference between the two antennas are in fig. S2. Rectification and subsequent regulation of the harvested voltage from Ant. 2 produce a constant 3.3-V voltage source for the heater. For a wide variety of smartphones, the root-mean-square (RMS) voltage harvested from Ant. 2 (V_rms) is greater than the threshold voltage (V_threshold) required for stable voltage regulator operation (Fig. 2D). Thus, the dual-coil powering scheme supports compatibility across a wide variety of smartphones.

The sensing component comprises two commercial surface-mount (SMT) thin film resistors (R = 680 ± 3.4 ohms each) connected in series to form a heater (~0.9 × 0.9 mm²) and an SMT negative temperature coefficient thermistor, placed 60 μm laterally away from the heater (Fig. 2B, subset). The heater can be switched on and off (see infrared camera image; Fig. 2E) by the μC. The change in temperature of the thermistor is captured by the analog-to-digital converter (ADC) of the μC and transmitted to the phone. Details of circuit operation and data transfer are in Materials and Methods. A bill of materials for all electronic components appears in table S1. The dynamic temperature range of the device is ~23° to 38°C, adjustable through the amplifier gain, with a minimum resolution of ~15 mK, limited by the ADC. Control experiments that use a wired system for power and data acquisition yield data that are indistinguishable from those captured using the NFC wireless system introduced here (fig. S2). As further validation, the SHS output for ΔT versus time (t) on standard materials with known k produces the expected, qualitatively inverse relationship between ΔT and k (Fig. 2F) (11). SHS measurements are calibrated against the ΔT versus t curve for water (which has known k = 0.6 W-K, α = 0.14 mm²/s). The results of measurements performed using n = 6 different sensors (5× each) with the same standard materials as shown in Fig. 2F appear normalized relative to the average measurement value for water at t = 13 s in Fig. 2G. Measurements of ΔT for a single device are highly repeatable, with an error of only ±2% (possible sources of variability are in fig. S2). Measurements of ΔT on curved substrates confirm that the device remains largely unaffected by mechanical deformation (fig. S3).

The sensitivity of the SHS (i.e., the range of ΔT for materials across the span of physiological values of k) depends, in part, on the fraction of the generated thermal power that passes through the sample of interest. Ideally, the thermal properties of the sample will dominate the response, ΔT. Alternate paths of thermal transport/thermal sinking, namely, those that can occur within the device itself, reduce the sensitivity to the sample. Associated techniques to improve sensitivity include reducing the widths and thicknesses of interconnect metal (Cu) traces in the vicinity of the heater/thermistor, reducing the thickness of the adhesive, and increasing the thermal power density (fig. S4). Sensor geometry can also alter measurement sensitivity; however, because of the small (~60 μm) spacing between the heater and thermistor, there is negligible difference in sensitivity between the case of a single component serving as both the heater and the thermistor and the separated heater/thermistor structure (fig. S5 and note S2). The distance between the heater and thermistor as well as the total measurement time determine the maximum measurement depth, which, for the configuration reported here, is ~1 mm (see note S2 and fig. S6 for details). Increasing the heater size and measurement time beyond the maximum of 13 s as is used in this work increases the measurement depth [~6-mm depth experimentally achieved in (7)].

Measuring skin water content

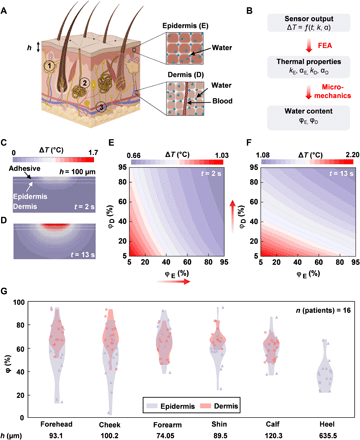
In previous reports, fitting procedures based on finite element analysis (FEA) and measurements of ΔT versus t define local, volumetric-averaged values of k for the sample under test, appropriate for homogeneous, isotropic materials (Fig. 2, F and G) (7, 9, 12, 13). Skin, however, is a layered structure that includes different features and heterogeneities. The outermost layer (i.e., the epidermis and its surface coating, known as the stratum corneum) contains water embedded within cells and in the tissue matrix. The dermis, the layer beneath the epidermis, contains this form of water as well as that associated with blood vessels (Fig. 3A). Modeling the skin as a two-layer system enables extraction of the averaged thermal properties of the epidermis and dermis, separately. FEA simulations relate the SHS measurements (ΔT versus t) to the thermal properties of skin. A micromechanics model treating the skin as a composite of dry tissue and water relates the thermal properties directly to the skin water content (φ) (Fig. 3B). The FEA model assumes a thickness h for the epidermis (based on body location; table S2), with an approximate value of ≈100 μm for most locations except for acral surfaces such as the heel (h ≈ 600 μm) and palm (14). At the microscale, the model for the epidermis consists of a composite of dry skin [k_dry = 0.2 W/(m-K), α_dry = 0.15 mm²/s] and water [k_W = 0.6 W/(m-K), α_W = 0.14 mm²/s] (12, 15). In this way, the equivalent thermal properties of the epidermis can be related to the volumetric epidermal water content φ_E according tokEkdry=(p+2)+2(p−1)φE(p+2)−(p−1)φE(1)αEαdry=αWkE(1−φE)αWkdry+φEαdrykW(2)where p=kWkdry, as plotted in fig. S7, and k_E and α_E correspond to the thermal conductivity and diffusivity of the epidermis, respectively. A similar simple model applies to the dermis, such that its equivalent thermal properties are given in Eqs. 1 and 2 with k_E, α_E, and φ_E replaced by k_D, α_D, and φ_D, respectively.

At the macroscale, FEA (see Materials and Methods for details) establishes a relationship between ΔT (at time t = 0 to 13 s) and the thermal properties k_E, α_E, k_D, and α_D, and, therefore, the water content φ_E and φ_D. For the typical epidermal thickness (h = 100 μm), at short times following initiation of heating (e.g., t = 2 s), thermal transport occurs substantially into the epidermis but only slightly into the dermis (Fig. 3C). Under these circumstances, ΔT is much more sensitive to φ_E than φ_D (Fig. 3E). Conversely, at long times (e.g., t = 13 s), the heat passes through the epidermis and considerably into the dermis (Fig. 3D). As the dermis is much thicker than the epidermis, ΔT in this regime is much more sensitive to φ_D than φ_E (Fig. 3F). This temporal separation allows separate determination of φ_E and φ_D from the ΔT versus t curves (see note S3 for details), thereby enabling real-time display of φ_E and φ_D on the phone application shortly after completing the measurement. For a few cases, depending on the individual and the body location, large epidermal thicknesses (e.g., heel, h ~ 600 μm) limit the transport of heat to the dermis even at t = 13 s. This behavior leads to a relative insensitivity of ΔT to φ_D (fig. S8), such that φ_D is indeterminate (14). In practice, for the cases reported here, errors in the values of φ_E and φ_D determined in this manner are <5% (fig. S9 and note S4). The main contributions to these errors are in the noise associated with the measurement of ΔT (< 3%) and in variations in h (<20%; note S4 and fig. S9). Measurements on a benchtop model consisting of mixtures of glycerin and water, prepared to span a wide range of physiologically relevant values of k, agree well with results from the FEA model developed here (fig. S4).

Measurements on several healthy/normal participants (n_patients = 16, demographics in table S3) on six different body locations each illustrate the accuracy of the fitting process and utility of the model (Fig. 3G; FEA fits with error values in fig. S10). φ_E has a larger variance than φ_D across the various body locations, attributed to the direct exposure of the epidermis to the environment and insulation of the dermis from environmental conditions. Furthermore, φ_E for the forehead and cheek show greater variations than that of other body locations, likely due to the presence of sebum, an oily, waxy substance excreted by sebaceous glands (with highest density on the face and scalp) largely comprising triglycerides, fatty acids, and wax esters, all which exhibit relatively low k [0.13 to 0.2 W/(m-K)] (16–19). Measurements across the various body locations yield a combined, average water content (including both φ_E and φ_D) for local areas (sans the heel) on healthy/normal participants of ~63%, consistent with the literature (6, 20). Typically, φ_D > φ_E (P = 0.0009, n_locations = 79; refer to Materials and Methods), as expected due to transepidermal water loss (TEWL) in the epidermis and the presence of blood vessels in the dermis. This finding agrees with reports in literature, which show lower average values of water content in the epidermis (~25 to 70%) than in the dermis (~70%) (21). These results validate that the model for skin hydration developed here is consistent with known skin physiology.

Measurements on patients with AD

Use of the SHS on patients diagnosed with various skin diseases yields a key set of results that illustrate the clinical utility and the versatility of the technology. For assessments of disease lesions, φ_E and φ_D, along with skin surface temperature T₀ are important indicators of different conditions, such as edema and erythema. We note that calculations of φ_E and φ_D involve measurements of ΔT and are thus independent of T₀. The same types of information can also serve as quantitative metrics of the efficacy of treatment strategies, for example, moisturizers/ointments. These combined features aid in diagnosis, treatment response tracking, and potential detection of flares. The following results also compare measurements of the SHS with those of a commercially available, portable moisture meter (gpskin barrier pro, GPower Inc., Korea) that determines both transepidermal water loss (TEWL; a contactless measurement using humidity and temperature sensors) and stratum corneum hydration (ScH; an electrical impedance/capacitive measurement), and shows good correlation to clinical gold standard Tewameter® and Corneometer® devices (22, 23). TEWL is a complementary measurement to skin hydration and assesses skin barrier function (6). We note that the aforementioned commercial skin impedance/capacitance-based devices typically involve only superficial measurement depths, confined to the stratum corneum (~15 μm) (24). Few devices have millimeter-scale measurement depths but have large (1 to 5 cm) probe diameters, which prevent small-area measurements (25).

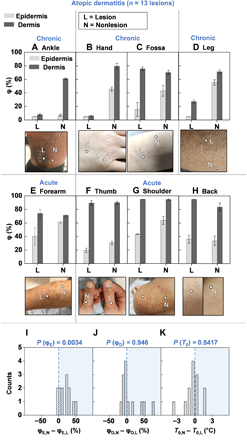
The first demonstration focuses on patients with AD, an inflammatory disease of the skin that causes red, itchy rashes on various locations of the body. In the United States, 18 million adults suffer from AD, with worldwide prevalence rates of 1 to 20% (26, 27). This pilot study involves n_lesion = 13 lesions across n_patient = 7 patients clinically diagnosed with AD by a dermatologist using both the SHS and the moisture meter. Patient demographics appear in table S4. SHS outputs consist of measurements of T₀, φ_E,L(N), and φ_D,L(N) on the diseased site (the lesion L) and on healthy-looking skin on a similar body location or perilesional area (nonlesional area N) as a standard of comparison. T₀ and moisture meter data for lesions in Fig. 4, not displayed in Fig. 4 for brevity, are in fig. S11. Results for all remaining lesions are in fig. S12. Three patients with chronic AD (Fig. 4, A to D) display low values of φ_E,L (<20%) compared with the nonlesional sites, consistent with the clinically dry appearances of the lesions. For the patients in Fig. 4 (A and B), the φ_D,L is also much lower at the site of the lesion than φ_D,N. Instances where φ_D,L < φ_D,N suggest that layers of skin below 100 μm are also drier than normal skin. A possible explanation is that the epidermis is thicker than the assumed value of 100 μm, attributed to hyperkeratosis. Figure 4 (E to H) displays images of acute AD lesions and their corresponding φ_E and φ_D. φ_E,L at the lesion is on average ~20% lower than φ_E,N (P = 0.0034). φ_D and T₀ do not display significant differences between the lesional and nonlesional sites (Fig. 4, I to K). These results are consistent with the understanding that AD is an epidermal disease. In contrast, data obtained with the moisture meter present no clear demarcation between diseased and nonlesional locations for ScH (P = 0.1099), but measurements of TEWL (P = 0.00024) (fig. S13) exhibit indications of the disease.

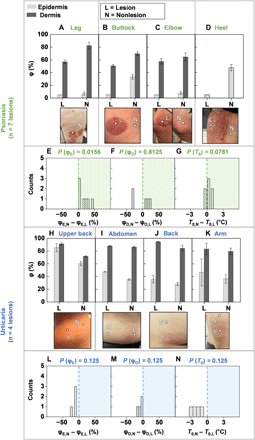
Measurements on patients with psoriasis and urticaria

Additional clinical studies use SHS to measure φ_E, φ_D, and T₀ (see FEA curve fits in fig. S14, and fig. S15 for T₀ and moisture meter data) for n_lesion = 7 lesions across n_patient = 3 patients with psoriasis—a skin disease characterized by a thickened epidermis and sanguineous, scaly, plaques affecting >8 million people in the United States (28, 29). For the psoriasis lesions presented in Fig. 5 (A to D), both φ_E,L and φ_E,N exhibit low values. As with certain patients with AD, φ_D,L < φ_D,N again suggest a thickening of the epidermis, which in the corresponding images appears as white, flaking skin. In other cases (fig. S13), φ_D,L is much larger than φ_D,N. Here, the lesions have a deep red color without the presence of peeling skin, suggesting a younger lesion with a thinner epidermis than the lesions in Fig. 5 (A to D). The color suggests elevated blood flow in the dermis. Hence, for older lesions, the SHS response is likely insensitive to this flow, whereas the sensitivity should be comparatively higher for younger lesions with relatively thinner epidermis. The value of φ_D, therefore, may lend insight into the age of psoriasis lesions, but this possibility merits further investigation on a larger number of patients. Of additional note are two unique cases of acral psoriasis (Fig. 5D and fig. S12), where φ_E,L < φ_E,N, consistent with the flaky dehydrated appearance of the lesion compared with the perilesional location. The SHS can differentiate between psoriasis lesions and nonlesional areas with φ_E (P = 0.0156). By contrast, differences in φ_D (P = 0.8125) and T₀ (P = 0.0781) are insignificant, confirming that psoriasis is an epidermal disease (Fig. 5, E to G). Measurements with the moisture meter also show some differences between the lesion and nonlesional areas (P = 0.0156 for both ScH and TEWL) (figs. S13 and S16).

The SHS can also provide insights into dermal diseases owing to its capability to measure properties deep into the skin, through measurements performed on patients with urticaria. This condition occurs in the epidermis and the upper portions of the dermis, where localized vasodilation occurs and biofluid exudes into the surrounding tissue (30). Measurements on n_patient = 2 patients for a total of n_lesion = 4 urticaria lesions indicate enhanced water content in the lesion than in the nonlesional area for both the epidermis and dermis (Fig. 5, H to M). The values of T₀ for all four lesions, furthermore, are consistently larger than those of the corresponding normal location, suggesting an expected enhancement of microvascular blood flow in the dermis of the lesions (Fig. 5N). Similar trends in T₀ also appear in lesions associated with a patient with a different dermal disease, rosacea (fig. S12). Because of the relatively small sample size, P is insignificant. The moisture meter exhibits no trend because of its shallow measurement depth (fig. S13). This collection of studies highlights the utility of SHS in assessing hydration across a broad range of skin diseases, with insights that extend beyond the superficial layers of skin compared with traditional skin impedance/TEWL–based devices. These findings correlate well with expected histopathology findings of both psoriasis and urticaria. The results demonstrate that the SHS can detect parameters that may serve as disease signatures—characteristic, disease-specific, quantitative trends in φ_E, φ_D, and/or T₀—for accurate, quantitative monitoring and detection of diverse dermatological conditions.

Topical treatment on a patient with xerosis cutis and AD

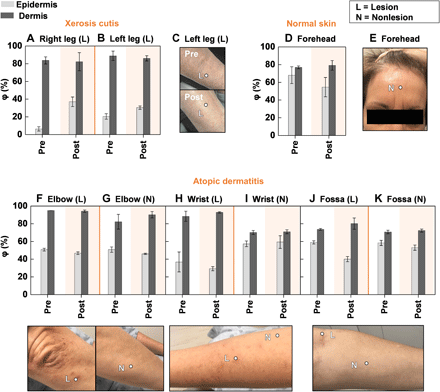
Diagnosing a disease state represents the first step in patient care. The next involves delivering treatment, monitoring the effects, modulating the treatment protocol as necessary, and potentially predicting for flares based on quantitative, individualized measurements on specific lesion sites. The results in the following demonstrate this approach in the context of a topical cream (CeraVe Moisturizing Cream, DFB Technology Ltd., USA) applied to a patient diagnosed with both xerosis cutis and AD.

Measurements with the SHS indicate that for both xerosis cutis lesions on the anterior leg, application of moisturizer for 30 min improves φ_E from its low initial value (+10 to 30%) but does not affect φ_D, as expected because moisturizers only improve superficial skin hydration (Fig. 6, A and B) (6). Photographs of the left leg of the participant before and after application of moisturizer visibly illustrate the improvement in hydration of the epidermis (Fig. 6C). Measurements of φ on the normal skin of the forehead before and after application of the moisturizer show negligible differences, as the skin of the forehead has high baseline hydration levels and a visually hydrated appearance (Fig. 6, D and E).

The acute AD lesions on the elbow, wrist, and antecubital fossa exhibit no increase in φ_E,L with moisturizer and negligible change in φ_D,L (Fig. 6, F to K). Results from a second patient treated with the same moisturizer on two AD lesions also indicate no observable changes in φ_E,L or φ_E,N (fig. S17). Because of the weeping nature of these acute AD lesions and because φ_E or φ_D indicates negligible differences between the lesion and nonlesional areas, this topical cream may not prove effective in improving the disease symptoms. Even for the same patient, moisturizer is effective only for certain diseases and locations. An important feature of the SHS measurement is that it probes deeply into the skin and can thus assess φ of the entire epidermis. In contrast, the moisture meter, as with other skin impedance/capacitance–based devices, has a shallow measurement depth, where measurements can be heavily influenced by the water content associated with residual moisturizer on the skin, not the skin itself. The SHS thus demonstrates potential in not only assisting in the diagnosis of skin disease but also in determining the effectiveness of topical and systemic treatments.

Study design

The objectives of this study were to design and demonstrate a robust, wireless, battery-free, and soft sensor for widespread, noninvasive monitoring of skin hydration independent of clinical settings, and to validate their performance against clinical standard tools. Patients recruited received full informed consent (Ann & Robert H. Lurie Children’s Hospital of Chicago, Chicago, IL; institutional review board study #STU00209010). Inclusion criteria specified healthy/normal participants or patients undergoing evaluation or routine checkups for clinical skin-related pathologies. A hypoallergenic silicone-based surgical tape (Kind Removal Silicone Tape, 3M Inc., USA) assisted in adhering the device to the skin. Sterilization of the moisture meters and SHS required individual wiping down of all surfaces with single-use alcohol wipes (Sterile Alcohol Prep Pads, Dynarex Corp., USA).

Fabrication of SHSs

Initial prototypes and proof-of-concept devices involved use of a laser cutter (LPKF U4, LPKF Inc., DE) to pattern a double-sided copper-clad laminate (Pyralux AP8535R, DuPont Inc., USA) and standard microsoldering techniques. To avoid damage to the critical sensing components, soldering the thermistor (NTCG063JF103FT, TDK Corporation, Japan) and heater resistors (RR0306P-681-D, Susumu Co. Ltd., Japan) using a low-temperature solder paste (TS391AX10, Chip Quik Inc., USA) and heat gun temperature of 200°C for less than 5 s was the final step in component assembly. Initial devices were prototyped in a laboratory setting, and the final designs were then sent to an external ISO-9001-compliant vendor for full manufacturing and assembly of multiple f-PCBs to illustrate the compatibility with readily available outsource manufacturing techniques. Programming the μC (RF430FRL152H, Texas Instruments Inc., USA) with a custom-designed embedded code using an NFC read/write interface (TRF7970AEVM, Texas Instruments Inc., USA) allowed for wireless communication of the sensor to a custom smartphone application. This software application features tunable measurement time and custom file naming.

Encapsulation of SHSs

The detailed fabrication procedures appear in the Supplementary Materials (fig. S1 and note S1). Briefly, preparing a layered structure of 45-μm thickness of silicone (Ecoflex 00-30, Smooth-On Inc., USA)/silicone gel (Ecoflex gel, Smooth-On Inc., USA), 30-μm-thin fiberglass fabric, followed by an additional 45-μm-thin silicone/silicone gel on a glass slide yielded the bottom layer of the SHS device, as the direct interface between the f-PCB and the skin. The f-PCB adhered to the silicone layer via bonding between a thin coating of SiO₂ sputter deposited onto the backside of the f-PCB and dangling ─OH bonds on the surface of the silicone (formed through ultraviolet-light functionalization). A custom-made aluminum mold and a hot press (Carver Press, Carver Inc., USA) allowed formation of a structured film of silicone (~2.7-mm thickness) as the top shell of the device. Screen printing uncured silicone beyond the outermost border of the f-PCB, placing the top shell and bottom layer together and subsequently curing of the entire device on a hotplate at 70°C for 10 min sealed the system. Cutting the completed structure out in a teardrop shape using a die cutter and peeling the structure off the glass slide completed the fabrication process.

Data collection and analysis

Powering the heater occurs through a regulated DC supply at a voltage of 3.3 V derived from the secondary AC voltage at Ant. 2, resulting in a constant thermal power of q = 10 mW/mm². The resistance of the heating element remains nearly constant due to its low temperature coefficient of resistance = 25 parts per million/°C. The thermistor connects as one of the arms of a Wheatstone bridge powered by a rectified 2.1 V from the RF μC. The voltage across the arms of the Wheatstone bridge is amplified, subsequently read by the RF μC ADC (sampling rate, 1 Hz; resolution, 10 bits), then transmitted to the phone (data rate, 0.125 Hz) NFC ISO15693 reader, and lastly recorded in the phone’s memory and also displayed on the screen. The application supports to the use of Android phones with NFC capability. The relationship between bit values and T appears in note S5.

Finite element analysis

FEA was performed using the commercial software ABAQUS. As shown in fig. S18, the model consists of all parts of the device related to the transfer including heater, thermistor, copper wires, PI substrate, Ecoflex adhesive, and measurement sample (epidermis and dermis). The air gap on top of the device is modeled by the adiabatic boundary condition at the top surface. A refined mesh much smaller than the finest feature size of the device (18 μm, copper thickness) was adopted to guarantee simulation convergence and accuracy. The material parameters used in all simulations are k_copper = 377 W/(m-K), α_copper = 109 mm²/s, k_PI = 0.12 W/(m-K), α_PI = 0.078 mm²/s, k_Ecoflex = 0.21 W/(m-K), and α_Ecoflex = 0.091 mm²/s, with k and α standing for thermal conductivity and thermal diffusivity, respectively (15, 31). FEA simulations on the influence of environmental temperature/humidity on the device appear in fig. S19.

Statistical analysis

All P values correspond to a two-sided Wilcoxon signed-rank test with the null hypothesis H₀ = N − L = 0. Error bars in various data plots indicate the SDs over three consecutive trials on the substrate/skin. Participants with dense coverage of hair and those who applied moisturizer were omitted from the data on healthy/normal participants in Fig. 3F.